, 
,
(3)
Ph.D. F.F. Gorbatsevich
There are quite a lot of works dedicated to revelation of the physical essence of the body inertia [1-4]. They present the historical development of the concept of “inertia”, various kinds of the body inertia manifestation and practical use of inertia in operating mechanisms. The notions of inertia and inertness are introduced. Some authors use the inertia and inertness notions designating one and the same body property by them. By inertia is most often meant the attempt of the body to preserve its state invariable in relation to the inertial (motionless) reference system. The most advanced formulation of the inertia properties is given in [5]: “Every body offers resistance in attempting to put it in motion or to change the modulus or direction of its movement”. Otherwise, if no external forces act on a body from other bodies or the environment, or these forces balance each other, the body preserves the state of rest or steady rectilinear motion. This expression is, in essence, Newton’s first law.
As is well known, Newton’s first law reads: every body is in the state of rest or steady rectilinear motion until the applied forces produce changes in this state [6]. Newton supposed that in the absolute vacuous space bodies – planets, stars, comets and other parts and particles of bodies can move freely. To a certain extent, such a situation assumes some “freedom of will”: after the primary push a body starts moving steadily and straight (as if without assistance) in space.
Experimental observations show that Newton’s first law can be obeyed only in some special abstract conditions deprived of the gravitational and other fields. It is well known that any space in the cosmos is filled with the gravitational field. Under gravitation the motion paths of cosmic bodies – comets, asteroids, planets, stars etc. are distorted and most often acquire elliptic orbits. In such an orbit the Moon travels about the Earth, the Earth and the Moon move round the Sun. The Sun together with the planets of the solar system travels around the galaxy centre with the velocity of about 250 km/s [7]. The motion of celestial as well as terrestrial bodies cannot be straight. Therefore the notions of the “straight motion” and “uniform motion” can be used only on certain assumptions, disregarding gravitational fields that penetrate the entire universe. On examining the motion of some terrestrial bodies in practice, one can concede that a body moves straight and uniformly on a certain short way where the curvature of its trajectory is moderately visible due to the gravitation effect.
On the other hand, it is intuitively obvious that if the gravitational force had disappeared all celestial bodies - comets, asteroids, planets, stars etc. would have started moving uniformly and straight, i.e. along straight lines in the outer space. In such a hypothetical space Newton’s first law would have been fully obeyed. A free motion along the straight path of the body would have changed only at collision with another body. That cluster of substance that represents a physical body has such an imperative property that if it is deprived of external forces it will preserve a straight uniform motion. This capacity is caused by the body’s mass. A moving physical body possesses kinetic energy besides its mass [4].
Unlike the power that a moving body possesses, motionless bodies can possess potential energy. But these bodies must be in some gradient force field, for instance, in a gravitational, magnetic or some other field. It was mentioned above that the entire outer space is penetrated by the vastest and most long-ranging field of gravitation forces. So all bodies that are limited in free motion possess potential energy. A body can be considered motionless if its potential energy is not realized in movement. If a body has no relations with other bodies that restrict its movement, its potential energy induces the body to move and turns into kinetic energy.
In classical mechanics developed by works of Galilee, Newton, D'Alembert, Lagrange, Euler et al. inertia of physical bodies is considered as a movement of a material point in relation to the coordinate system, motionless (absolute) or mobile, for instance, related to planet Earth. Within the approach using the motionless coordinate system, D'Alembert (in studies of the movement of a constrained point) introduced vector D in mechanics equal to the product of the mass m of a point body by its acceleration a taken with the opposite sign [2],
|
D = - ma, | (1) |
This vector was later called d’Alembert’s force. According to Newton’s third law, this force must balance the force accelerating a body. On this approach, the interaction of the force and body mass described by Newton’s second law can be reduced to a static task. A more general principle that allows solving complex equations of dynamics is called D’Alembert-Lagrange principle: “In motion of the system with ideal relations (i.e. such relations which reactions cannot produce work – without friction, losses of energy and these relations) at every instant time the total elementary work of all active forces applied (i.e. not reactions) and all inertia forces at any possible movement of the system will be equal to zero” [4]. This principle allows setting up an equation of motion for any mechanical system and finding the forces acting inside the system.
For the mobile coordinate system relative to some “absolute”, great Euler offered additional inertia forces – transportable P and coriolis Q [8]. The complete dynamics equation of a point body with regard for these inertia forces takes the form:
|
ma = F + P + Q, | (2) |
where F is an acting physical force.
Interpretation of the notion of “force” at present is not unequivocal. As is considered in mechanics [3], Eq. (2) has the structure of the initial equation of absolute motion in which the terms P and Q have a force dimension. But in the author’s opinion [3], these terms do not produce any mechanical effect; therefore they are not physical forces. These forces are considered to be inertia forces. A force is the vector measure of mechanical manifestation of bodies’ interaction. Periodically some controversy arises among specialists in classic mechanics as to whether the forces P and Q are real or not. The same doubt appears about d’Alembert’s force D. Most likely these forces are the body’s responses to the effort to change its state in accordance with Newton’s first law. These forces are considered to be inertia forces. It has been suggested that the forces that are exhibited during a mechanical contact between bodies, should be called physical forces.
In our opinion, some general interpretation of the “inertia” concept should be formulated. Such an interpretation or determination can be found when analyzing the situations in which inertia is immediately manifested when bodies interact.
The simplest way is to consider the inertia and energy of two impinging bodies. The body inertia is known to be proportional to its mass; it greatly increases with increasing the body velocity. Let us consider a perfectly inelastic impact using the collision of balls as an example [5]. Assume that the balls move along the straight line connecting their centres with velocities V1 and V2. In this case the impact is considered to be central. Let us denote the general velocity of the balls after the collision by V. The kinetic energies of the system before the impact
Ê1 and after the impact Ê2 will be respectively:|
| (3) |
where m1 and m2 are the masses of the balls.
The difference
is the part of energy that was absorbed at collision. At nonelastic collision of two identical bodies approaching each other with the same speed, their mutual speed after the collision is equal to zero and the entire energy is spent for breaking-down the bodies (for instance, two cars). At the perfectly elastic impact the internal energy of bodies does not change [5], i.e. the total energy of every body before the impact is equal to the total energy of these bodies after the impact.
It is most simple to calculate the quantitative characteristic of the body inertia estimating its change at collision of a small body with a body of a much greater mass M. Let us estimate the energy that will be released at this collision. From (3) it can be derived that if mass m of one body is much less than the mass of the other body (V = V2 = 0), the kinetic energy released at impact will be equal to (if the second-order effects are disregarded):
|
| (4) |
This formula was first obtained by Oliver Heaviside and independently of him by William Thomson [9]. From this formula it follows that at collision the entire energy released at the cost of the small body. The body with mass m moving at a speed of V after the collision with the body with mass M will have the speed V = 0. After the collision the small body lost all inertia in relation to the body M. According to Eq. (4), its motion energy will be equal to zero. This example shows that the body inertia can be set equal to the amount of kinetic energy accumulated in its mass and speed.
The above allows the conclusion that the inertia or kinetic energy of a body can be estimated only in relation to another body relative to which it moves. If no abstract coordinate system is introduced then inertia as well as kinetic energy is inherent to the body that can be considered to be moving relative to another body. Within the assumption of a possibility for uniform straight motion one should realize the relativity of notions of “motionless” and “moving” bodies. For instance, a body does not possess inertia in relation to another body relative to which it does not move. When a body is at rest in relation to another body it does not possess inertia in relation to that other body. One can envision two bodies moving in one direction with the same speed. They will not possess inertia in relation to each other. But these two bodies will possess inertia in relation to a third body moving relative to them with some speed different from zero.
Therefore the inertia (potential) measure of every specific body moving independently has a great number of values depending on the fact in relation to which other body it is estimated. The inertia of one and the same body or its kinetic power will be different in relation to bodies moving with various speeds. It is exhibited only at collision with another body. Some external object is necessary to estimate the inertia measure or for the inertia of a physical body to be mechanically manifested. So this type of inertia should be called external inertia.
Unlike a free inertial body which movement can be (conventionally) estimated as uniform and straight, a body rotating around some axis possesses a special type of inertia. This type can be considered to be internal inertia of the body. Internal inertia and its estimation do not depend on external bodies. It has one certain
meaning. Internal inertia and the body rotational energy are necessarily accompanied by stresses and strains inside the body at the cost of appearance of centrifugal forces. Internal inertia is accompanied by the gyroscopic effect. The centripetal force equal to the centrifugal one ensures stability in the body rotation. Since the centripetal force is constantly applied to a moving body, then, according to Newton’s second law, the body is considered to be in accelerated motion. At the same time the body moves uniformly, i.e. the linear velocity of its every individual point remains constant. Since this body moves uniformly, then, according to Newton’s first law, one can assume that it moves without acceleration. Most likely, the last mentioned statement has more reasons since the body quantitative characteristics do not change when it is in uniform circular motion. The motion energy and the body inertia remain constant.There is a strict correspondence between the body mass, the angular velocity of its rotation, internal stresses and strains. The potential inertia of a rotating body can be numerically estimated by the moment of inertia. The moment of inertia of a material point relative to some axis is equal to the product of its mass by the squared distance from the point to this axis [6]:
|
J = mR2 | (5) |
The moment of the body inertia is the sum of the moments of inertia of material points constituting this body. The kinetic energy of a rotating body is equal to [6]:
|
| (6) |
where ω is the angular velocity, rad.
Planet Earth rotating round its axis has internal inertia. Due to this inertia the geoid shape, in the first approximation, represents a flattened ellipsoid which bigger radius is located in the equator plane. The Earth-Moon and Sun-Earth systems have internal inertia as every pair star-planet. The body internal inertia can be found experimentally. For instance, measurements of equatorial and polar radii of the globe reveal deformations of the Earth shape due to its rotation round its axis. Coriolis force (see expression (2)) deflecting the threads of streams westward in the north hemisphere and eastward in the southern one points to the manifestation of the Earth’s internal inertia. Indoors Foucault pendulum clearly demonstrates the presence of this internal inertia. Since one can reveal the fact of a body rotation in an isolated space experimentally, Galilean relativity principle is inapplicable for rotating bodies. Thus, strictly speaking, Galilean principle cannot be followed under the earthly conditions.
One can give a lot of vivid examples of a system of bodies that have great internal inertia. One of them is the solar-planetary system. In it the planets move along the elliptic orbits close to circular ones. This movement is possible only with the constant centripetal force Fc applied to the moving body in the direction perpendicular to the motion direction. During a circular movement this force is applied from one centre (a simple case). To make this body move along the circular trajectory this force should be equal to [6]:
|
| (7) |
where R is radius, m is mass, V is linear velocity of the body movement along the orbit.
Concerning the body that is on the earth orbit, the role of force Fc is played by the earth gravity.
Within mechanics determined by Newton’s first and second laws, besides inertia the second characteristic that closely related to it should be singled out, i.e. inertness. Inertness and inertia are different categories. A number of well-known works [1-4] indicate that the inertness measure of a body is its mass. “By convention the mass of an elementary particle is determined by the fields related to it – electromagnetic, nuclear etc., but the quantitative theory of mass has not been created so far” [4]. The differences in masses of various bodies are revealed in attempting to change their state, for instance, to accelerate or decelerate, to change the direction of their movement.
Newton’s second law establishes the measure of the body mass (for simplicity of consideration we do not take into account the vector nature of the force and acceleration):
|
F = ma, | (8) |
where F is the force inducing the body to change its state, a – is the acceleration that the body acquires. Newton’s second law shows that with one and the same force F, the greater is the mass m, the less is the acceleration acquired by the body.
Inertness is inherent in every body that possesses mass. Inertness is the property of physical bodies to show resistance in attempting to set them in motion, to change the modulus or direction of this motion. Thus, inertness is also inherent in motionless bodies. Inertness, as well as mass, determines the ability of a body to move freely in a free space. According to Newton’s second law the categories of inertness and mass mean one and the same property of physical bodies. In this connection the category “inertness” can be convincingly considered to be a synonym to the category “mass”.
As mentioned above, unlike inertness the body inertia is related to its movement. There are two main kinds of inertia – external and internal. External inertia is the inertia of a body moving (within some assumptions) uniformly and linearly. The inertia of a moving body does not manifest itself in any way until it collides with another body.
Internal inertia is inherent in rotating bodies. Unlike bodies possessing external inertia, bodies possessing internal inertia experience internal strain and stress. If a rotating body is deprived of external relations it will rotate for a long time without limit maintaining one and the same rotation energy.
These notions of internal and external inertia have long been known in one form or another. Even Galileo differentiated these kinds of inertia. In other formulations they can be interpreted as “rotatory” and “linear” inertia. In our opinion, a clear separation of the inertia types into internal and external, an emphasis on distinction between inertia and inertness will provide better insight into the essence of these very important physical categories. On the other hand, the
equality of inertness and mass of a body has long been accepted in classical mechanics [3]. In our opinion, a detailed analysis of these notions presented above is necessary to gain a clearer understanding of their physical essence.On the basis of the above mentioned it can be noted that the first Newton law characterizes the rest and motion of the free body that has, within our definitions, only external inertia. It is unsuitable for characterizing the internal inertia. In this connection the demand arises for formulating the combining law that would characterize both types of inertia. In our mind, this formulation might look as follows: “a body set in motion that is free from external relations will move indefinitely long conserving the motion energy”. This formulation combines both types of inertia. It is fuller and it can be called the law of inertia.
Consider one more aspect related to inertness or body mass. The problem of the difference between gravitating and inert masses has long been discussed in Newton laws of motion. Some physicists believe that these masses can be different. A lot of thin and rather precise experiments were performed with the aim to find the difference between gravitating and inert masses [10-13]. This difference has not been found so far [5]. On the basis of the approach discussed above it can be stated that this difference must not exist since inertness and mass are adequate categories.
Imagine that the force Ft is acting on some inert mass according to Newton’s second law (8) and acquires the acceleration a which value is inversely proportional to the body mass m. The gravitational field acts on the mass m as some force Fg. This force arises because the gravitational field (of the Earth, Sun, intergalactic field etc.) is gradient [14]. When a body of one and the same mass m acquires acceleration by the force Ft or the gravitational force Fg
, the forces and acceleration of the body can be variables, but the mass cannot. The well known experiments of physicist R. Eötvös prove only the fact that bodies possessing any physical properties and masses move in a gravitation field in a similar way [10, 15]. Thus, the inert and gravitational masses are equal since they have one and the same basis – the body inertness or, otherwise, the effort of the body to maintain its state in attempting to change this state.At the same time, peculiarities of the force application during acceleration of the body in the gravitational field and through the contact with another body differ greatly. As mentioned above, every motionless body (for instance, lying on a support) in the gravitational field possesses potential energy. A motionless body in the gravitational field experiences internal loads and strains.
To show this let us divide the motionless body on the
support into several parts. The parts that are lying nearer to the support experience greater loads than the overlying ones due to the pressure coming from the overlying parts (the load on our feet is greater than that on our shoulders). Our soles undergo the pressure equal to the body weight. The pressure perception in our body is the ponderability perception. Internal loads and strains also arise in the body that is accelerated by some force. This body can also be divided into several parts. It will turn out that the parts located near the point (surface) of the accelerating force application experience the greatest internal loads and strains. In such bodies forces (causing acceleration) are applied locally, i.e. at a point, plane or surface.Imagine a free-moving closed capsule (lift) in which some physical body is situated. Does the gravitational field inside this capsule disappear or decrease? It would happen if the capsule velocity was greater than the propagation velocity of the gravitational field. As experience shows, gravitation is not virtually shielded by other (not very massive) bodies. So the gradient field of gravitation will accelerate the body in the capsule and the capsule itself with equal velocity.
The Great Galileo was the first to show that light and heavy bodies fall with equal acceleration in the Earth gravity field. The most pictorial experiment demonstrates a fall of a lead pellet and bird feather in a vacuumized glass tube. It is clearly seen that both things fall with equal acceleration. If we divide some body into pieces and follow the fall of the pieces in the vacuum, we shall see that both big and small pieces fall with equal acceleration in the Earth gravity field. If we continue the division up to atoms we shall see the same result. Hence it follows that a gravitational field is applied to every elementary particle possessing mass and constituting a physical body. It will accelerate big and small bodies with equal velocity only if the field is gradient and acts on every elementary particle of the body.
A gravitational field is really gradient. The force action on an elementary particle can be compared with the liquid pressure on the body submerged into it [16]. Such an interpretation allows explaining the ‘zero-gravity” state that a freely falling body experiences. In classical mechanics the zero-gravity perception is interpreted in the following way [3]: “What is zero-gravity and how should it be understood from the viewpoint of mechanics? First of all note the following. The human nervous system delivers information to the brain about how one part of the body weighs upon another…” “If the surface forces are absent, for instance, when we fall from a height before the parachute opens (air resistance is disregarded), then the pressure of the body’s one part on another is missing and the zero-gravity perception arises.”
In a free fall a physical body starts moving in the direction of the field gradient decrease. If we divide this body into some separate parts (particles) it will appear that equal gradient pressure is applied to every one of these particles (if the gradient change within the body length is disregarded). We showed above that both large and small (and minute) bodies are accelerated with equal velocity in the gravitational field. Hence it follows that the gravitational pressure is distributed in such a way that it is applied to every atom (including its nucleus and electrons) constituting the body. The gravitational pressure is not applied to the body as a whole at some point or surface; it is distributed and acts inside the body. Every part and every atom gets equal acceleration in the gravitational field. Since accelerations gained by the parts (particles) constituting the body are equal, stresses and strains are missing between these parts and the state that is called weightlessness is realized. Weightlessness is exactly the state when internal stresses between the body parts are missing (if, for instance, the long bar oriented in the direction of the gravity gradient is not considered).
Note that unlike the body that rests on the support and is in the gravitational field, the gravitational force in the body that is free from external relations is not applied locally, but acts equally on every particle constituting the body. In this state gravitation is responsible for acceleration, an increase in the body velocity in the free motion to the centre of gravity. If the force applied to the body through the contact point or surface is the vector measure of mechanical manifestation of the bodies’ interaction, then the gravitational force is the distributed gradient pressure on every elementary particle constituting the body.
Thus, the gravitation activity is possible due to the fact that the gravitational field is a gradient one and its gradient is applied not to some point of the body or its part or to the body as a whole, but to every elementary particle constituting this body. But in order that the gradient field delivering its gradient pressure to every elementary particle should exist, a medium freely penetrating into all physical bodies without exception must be available. Only in this case the principle of close-range interaction will be observed. Such a medium that penetrates into all physical bodies freely and exerts gradient pressure of gravitation is ether or ether medium [16]. The gravitation action through the ether medium disappears neither in the body lying motionlessly on the support nor in the body that is in a free fall. The gradient pressure of the ether medium in the body lying motionlessly on the support acts through forces on every elementary particle. These forces add up together to give a resultant pressure of the body on the support. We call this pressure the body weight. Deformations occur inside the body. The closer is the area under consideration inside the body to the support the greater are the deformations.
In the free fall the gradient pressure of the ether medium is distributed inside the body. It acts on every elementary particle inside the body and its value remains the same as in the motionless body. The gradient pressure of the ether appears because the sizes of the ether particles are estimated to be much less than those of the elementary particles constituting physical bodies. Analysing the effective polarizability of the ether particles A.A. Potapov [17] arrived at the conclusion that their size is equal to Planckian length:
a = lp = 
= ~ 1·10-32 mm,
where h is Planck constant, G is gravitational constant,
Ñ – is light velocity.This size is several orders less than the size of elementary particles. As is well known, atom sizes including their electron shells are fractions and units of angstroms, i.e. ~ (1-10)
× 10-10 m. Nucleus sizes are z~10-14 m. The electron radius (classical) is 2.818× 10-15 m [18]. The sizes of other elementary particles are not too different from those of electrons and can be no more than two orders less. If we compare the sizes of the ether particles (~ 1·10-35 m), nuclei and an electron we shall see that the ether particles can easily penetrate and take places among atoms in the intra-atomic space. The light penetration into transparent bodies (the wave length, for instance, of the visible light is (4-7)10-7 m [19]) corroborates the sameness of the base which oscillates being excited by the light quanta both in the vacuum and inside the bodies [20]. A physical body composed of atom nuclei and electrons represents a kind of a space “lattice” inside of which the ether is situated. Its gradient pressure on nuclei and electrons constituting this “lattice” gives rise to the gravitation force. From the above it follows that the ether medium is an essential subject of classical mechanics and cannot be excluded from it. The presence of the ether medium allows the nature of gravity to be explained [16]. At the same time there are concepts denying the existence of the ether medium. At present many tens of the gravitation theory versions have been suggested [21]. One of the best known is set forth in the general theory of relativity (GRT). According to this theory there is a correlation between gravitation and inertia. This correlation is explained by the fact that gravitation and inertia seem to be the same thing because they are the same thing. In the GRT this correlation is called the equivalence principle. According to A. Einstein’s letter to E. Mach, the equivalence principle lies in the equivalence of the accelerated system and gravitational field ([22], p.169). In other words, by this theory, in a lift moving in the cosmos with acceleration exactly equal to the acceleration of the body falling to the Earth, a force equal to the earth gravity will be created artificially. It is reasonable that this imaginary cosmos should be devoid of the gravitational field.It can be shown with relative ease that there is a fundamental difference between the state of the body that is in the gravitational field with the gravitation constant G and the state of the body speeded up with the same acceleration F = G. Let us make up a body consisting of two masses united by bars as shown in Fig. 1a. Let us build the dynamometer D1 into the horizontal bar. Let us make up the second body with the same composition as the first one with the dynamometer D2, Fig. 1b. The first body is speeded up by the force F with acceleration equal to G in the space unable to display the gravity force. The second body is in stable equilibrium in the gravitational field with the acceleration G. It is easy to notice that the readings of dynamometer D1 on the body of Fig 1a will be greater than those of dynamometer D2 on the body of Fig. 1b since the body masses in Fig. 1b will be attracted together. This example clearly shows the inconsistency of the equivalence principle. But this example demonstrates only the correctness of Newton’s second law. When we imitate gravitation in the body that is in the lift moving up, the distributed gravitational field is absent.
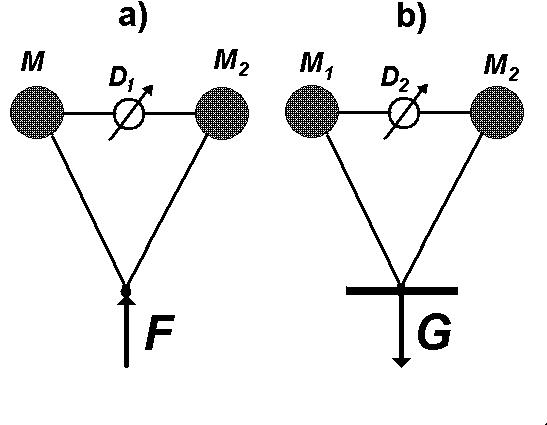
Fig. 1. The diagram showing the difference between the state of the body accelerated by a physical force (a). and that of the immobile body in the gravitational field (b).
It is easy to explain the formation mechanism of the weightlessness state inside the satellite moving on the near-Earth orbit with our concept of the gradient gravitational field. As applied to the body situated on the Earth orbit, the Earth gravity plays the role of the force Fc (Eq. (7)). In this case Fc = mg, where g – is the acceleration of the gravity force. The average value of the earth acceleration g = 9.81 m/s2. Substituting the value of the centripetal force that ensures the value of the earth gravity in Eq. 7 we obtain that in the Earth-circling orbit (excluding the influence of the Earth form, the Moon, the Sun etc),
g = V2/R.(9)
In this case the centripetal force caused by the Earth gravity will be balanced by the centrifugal force of the inertia Fc similar to Euler transportable force
Ð [8]. The centrifugal force Fc, as well as the gravitational force, acts equally on every part, particle and atom of the orbital physical body balancing the gravitational force. Therein lies the cause for the zero-gravity of bodies in the Earth orbit. Note that according to Eq. (9), all bodies independently of their mass that have reached the circular velocity V, will become “weightless”.As a result note that it is just the gravitational force representing the gradient pressure on every elementary particle constituting a physical body that causes all bodies to fall with equal acceleration. A great scientist Galileo Galilei was the first to establish this fact. Accepting the concept that gravitation is the distributed gradient pressure resolves the problem of identity of gravitating and inert masses.
The above arguments show the striking fact that some problems of not only celestial but also classical mechanics cannot be solved without the ether concept. It is appropriate at this point to cite mechanical engineer A. Ishlinsky [3]: “Are inertia forces real or unreal? Supporters of the inertia force reality advance the facts of a cord tension as a stone rotates on it, a rupture of fast-revolving flywheels, a feeling of additional gravity in a lift going up etc. They state we would not be able to distinguish the inertia forces from “real” ones if we always were underground and knew nothing about the Earth rotation around its axis and its movement around the Sun. Would we come to Galileo-Newton ordinary laws of motion in this case? One can bring forward a large number of such examples and questions”. As indicated above, the acceptance of the ether medium concept allows removing the existing contradictions.
In a general evaluation of the inertial motion one should take into account that all bodies move in the ether or otherwise in the ether medium [14]. This refers to both cosmic bodies and microparticles. The above assessment of the body inertia pertains to the values of the motion velocity that are much lower than the velocities of electromagnetic waves (light) in the ether C. A body moving in the ether medium with the velocity that is much lower than the light velocity virtually does not interact with the ether medium [18]. A physical body moving uniformly in the ether medium does not experience resistance. The ether resembles an ideal medium displaying no friction effects. D’Alembert pointed to the possibility of a body to maintain the uniform and straight motion in an ideal liquid experiencing no resistance at all [2]. But at the same time the ether has certain characteristics like a specific solid body [14].
Revealing the peculiarities and properties of the ether medium has been taking place for a very long period of time. The first assumptions about the existence of the luminiferous and all pervasive medium were formulated in ancient Greece. Newton considered the ether to be responsible for transmission of gravitational effects: “To suppose that one body can act on the other one that is at some distance from it through vacuum without any “moderator”…- is so absurd to me that, to my mind, not a single person that has the slightest idea of philosophical matters can believe this” [1]. James Clerk Maxwell revealed the distinction of the ether from the well-known media. “The ether differs from ordinary matter. When light moves through the air then it is obvious that the medium through which the light travels is not the air itself, because, first, the air cannot transmit shear waves and compression waves transmitted by it propagate a million times slower than light”… “Whatever difficulties we faced attempting to elaborate a notion of the ether structure, but undoubtedly, the interplanetary and interstellar space are not empty spaces but filled with a material substance or body that is the vastest and most homogenous known body” [23 (Reversed translation from Russian)].
In what follows the discovery of electromagnetic waves by G. Hertz attached some new very important properties to the ether. Besides transmission of gravitational effects, the influence of static electric charges, force of magnets, the ether can transmit electromagnetic waves. It possesses dielectric and magnetic permeability, wave resistance, specific density. The sunlight appeared to be a set of electromagnetic waves. Infrared and ultraviolet rays, hard X-rays are transmitted by the same carrier – the ether or, put otherwise, the ether medium.
More recently by observation results of the Earth-geostationary satellite system it was corroborated that the electromagnetic wave velocity in the free space is determined only by the ether medium properties [24]. No matter with what velocity the source may move, the velocity of electromagnetic waves from it is equal to the speed of light in the ether. In this case the influence of the Earth movement on the aberration of the electromagnetic waves from the source placed on the satellite was found. This allowed one to measure the parameters of the absolute movement of the Earth and solar system without astronomic stellar observations. The obtained values of the orbital component of the Earth velocity (29.4 km/s), right ascension of the Earth apex (270
î) and its declination (89.5î), as well as the velocities of the absolute movement of the Solar system agree with the well-known ones in the observational astronomy. This result is a direct proof of the fact that the velocity of the uniformly moving laboratory coordinate system (in our case the Earth) can be actually measured with the device in which the radiator source (geostationary satellite) and receiver (antenna of a terrestrial telescope) are at rest relative to each other and to the same coordinate system. That is to say that the ether medium in the interstellar space can be taken as the independent coordinate system.The interaction of a physical body (particle) moving with the near-light speed is most conspicuous in the phenomenon called Cherenkov effect. This effect was first registered in transparent water surrounding radioactive materials emitting high-energy particles. The effect lies in emission of the light quanta by a particle if the particle, for instance, an electron moves with the speed V exceeding the light phase velocity Vf in this medium. In this case the following condition is met [25]:
, (10)
where
Ñ is the speed of light in the vacuum (ether medium), n is the refraction index at the frequency ω. This effect lies in the fact that a particle emits light within a certain dispersion cone. The cone angle θ is determined by the relation between the phase speed of light in the medium and the movement of a charged particle in it,
(11)
The relations (10) and (11) were obtained on the basis of analogies with the effects arising when bodies move in gases with the speed close to the speed of sound in gas. V.L. Ginzburg [25] pointed that such analogies are useful: “Analogies, a transfer of notions from one field into another, are of great importance in the development of physics and (there is little doubt about it) other sciences. Therefore for fruitful work in science it is very important to have a wide outlook and not to limit oneself by a narrow specialization as is often the case”. The cone of the arising emission (11) is similar to Mach cone that restricts the front of the shock wave in the gas medium (air) when a body moves with the supersonic speed. A shock wave in gas arises as the velocity of the body exceeds the speed of sound, i.e. when the condition (10) is met.
Cherenkov effect has been registered in transparent media in which the velocity of electromagnetic waves is lower than the speed of these waves in the ether. There are some works in which the motion of particles with supraluminal speeds has been registered experimentally [26]. In the experiments Cherenkov radiation in the motion of the lead ion beam was observed. The speed of the particles was found to exceed the light speed by (1.00006-1.0047)
Ñ. There is A. Zommerfeld’s work in which the motion of a charge in the vacuum (i.e. in the ether) with the supraluminal speed is considered [27]. The author obtained the result that such a charge must radiate.So the particle moving with the near-light speed radiates. This means that it looses the motion energy and inertia. Thus, the way of this particle travelling faster than light is finite. Besides, the mass of the moving particle (body) depends on the speed value, if this speed approaches the light speed, i.e. the speed with which electromagnetic oscillations propagate in the ether. Numerous experiments show that the body mass depends on the velocity of its travel in the ether medium. This dependence is expressed by the following formula [22]:
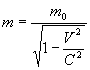, (12)
where m0 is the mass of the particle at rest, m is the mass of the particle moving in relation to the ether medium, V is the particle velocity,
Ñ is the speed of light.The dependence (12) shows that as the particle speed approaches the light speed one can observe the phenomena similar to those arising when the body speed approaches the sound speed in gases. It is also useful here to apply the analogue method mentioned by V.L. Ginzburg. But the fundamental difference exists between these phenomena. As a body moves in gas the gas flows round it. When moving in the ether medium, the ether medium moves through the physical body [20]. In this case the ether medium interacts immediately with every elementary particle constituting the physical body and possessing mass – with electrons, protons, neutrons etc.
As the particle speed approaches the light speed its mass becomes equal to m (12). If we slow down this particle its speed again becomes equal to m0 – i.e. to the mass at rest. Most likely it means that the mass of the particle itself independently of its speed in the ether is equal to m0. The additional mass equal to m
å = m - m0 is the associated mass of the ether.The formula (12) allows the conclusion that a motion in the ether medium is possible with any minor difference between V and C. But this motion cannot last for a long time with the speed V = C, since in this case the energy and mass of the particle will be infinitely great. The mass-energy conservation law puts infinitely great masses of any bodies under ban. This suggests that physical bodies cannot move through the ether medium with the light speed for a long time.
The last mentioned conclusion has an important consequence – photons, light quanta that always propagate with the velocity C cannot have mass and consequently, they are the waves perturbing the ether medium and propagating in it. As is known, photons (quanta) have a wide spectrum of varying frequencies, from the thermal range to X-rays. An infinite number of photons (quanta) of different frequencies propagate in the ether in all directions and are received by devices as electromagnetic waves, light and X-rays.
In conclusion, summing up some results, note dissimilarities of the inertia (kinetic energy) of bodies moving with the speed much lower than the light speed (low speeds) from that of the bodies moving with the near-light speed. As to the bodies moving with low speeds, one can note that a body does not possess inertia in relation to the other body relative to which it does not move. The greater is the mass and speed of a body, the higher is its inertia in relation to another body. The body inertia will be different in relation to other bodies moving with varying speeds. Therefore the motion inertia of every specific body has a lot of values depending on the fact in relation to what other body it is assessed.
As bodies (particles) move with the near-light speed Newton’s laws are broken. The moving bodies interact with the ether. Their inertia substantially increases. Just as in the motion with low speeds, the body inertia can be assessed in relation to another body. But in the motion with the near-light speed the body inertia can be also assessed in relation to the ether medium itself. In our opinion, in the motion with the near-light speed part of the ether mass carried along joins the body mass. The movement of bodies in the ether cannot last long with the speed equal to the speed of electromagnetic waves in the ether, since in this case the inertia, energy and mass of the particle will become infinitely great.
Finally note that the separation of the notions related to mass (inertness) and motion (inertia) of a physical body makes these fundamental categories clearer and more explainable. The so-called “mystery of the inertia forces” disappears. From this viewpoint Mach principle [28] – “the inertia force of any body is caused by its gravitational interaction with all remote masses of the universe” is also subject to criticism. Due to assessment variability of the inertia force including that of cosmic bodies in relation to every other, this principle becomes not obvious. At the same time owing to the equivalence of the notions of “inertness’ and “mass” and some properties of the ether one should state that the gravitation force at any specific point of the universe is determined by all cosmic masses contained in the universe. These masses include all visible and invisible forms wherever they were. In the context of the ether medium theory the last mentioned statement is reliable. The ether medium transfers the gravitational action (as well as other field types) from one physical body to another. Every physical body distorts the ether medium that is in its vicinity. The attracting force decreases inversely with the squared distance from a body of the spherical shape. It becomes weaker with distance rapidly. At the same time due to the ether medium this force does not cease its action at any, even astronomically great, distance.
1. Newton. Mathematical principles of natural philosophy. Optics. Britannica, V. 32. Second Edition. Chicago. 1994.
2. D’Alembert, Jean Le Rhone. Dynamics. Novokuznetsk: IO NFMI, 2000. 336 p. (in Russian).
3. Ishlinsky, A.Yu. Mechanics of relative motion and inertia force. Moscow, Nauka, 1981. 320 p. (in Russian).
4. Gulia, N.V. Inertia. Moscow, Nauka, 1982. 152 p. (in Russian).
5. Sivukhin, D.V. General course of physics. Vol. 1. Mechanics. Moscow, FIZMATLIT, 2006. 560 p. (in Russian).
6. Koshkin, N.I. and Shirkevich, M.G. Handbook of elementary physics. Moscow, Nauka, 1974. 225 p. (in Russian).
7. Kulikova, V.V., Kulikov, V.S., Bychkova, Ya.V. and Bychkov, A.Yu. The Earth history in galactic and solar cycles. Petrozavodsk, Acad. Sciences, 2005. 250 p. (in Russian).
8. Euler, L. Fundamentals of the point dynamics. M.-L., Gl. Red. tekhn.-teor. lit., 1938. 502 p. (in Russian).
9. Thomson, W. (Lord Kelvin) Mathematical and Physical Papers. Vol. III, Art. XCIX (49), C (50), CII (52). London, Cambridge University Press, 1890. 529 p.
10. Eötvös, R., Pekar, D. and Fekete, E. Beiträge zur Gezetze der Proportionalität von Tragheit und Gravität. Annalen der Phys., 68, 1922. P. 1-56.
11. Roll, P.G., Krotkov, R. and Dicke, R.H. Ann. Phys. 26, 442, 1964.
12. Sagitov, M.U. Gravitation constant and the Earth mass. Moscow, FIZMATGIZ, Nauka, 1969. 188 p. (in Russian).
13. Braginsky, V.B. Physical experiments with test bodies. Moscow, Nauka, 1970. 136 p. (in Russian).
14. Gorbatsevich, F.F. Theoretical fundamentals of non-empty ether. Apatity, MILORI Publ., 1998. 48 p. (in Russian).
15. Grushinsky, N.P. and Grushinsky, A.N. In the world of gravitational forces. Moscow, Nedra, 1978. 175 p. (in Russian).
16. Gorbatsevich, F.F. On gravity force. In: Space, time, gravity. Proc. of IX Int. Conf. 7-11 August 2006, St. Petersburg. St. Petersburg, “TESSA”, 2007. P. 86-97. (in Russian).
17. Potapov, A.A. Deformation polarization. Search for optimal models. Novosibirsk, Nauka, 2004. 511 p. (in Russian).
18. Ebert, H. Physikalisches Taschenbuch. Zweite Auflage. Friedr. Vieweg & Sohn, Braunschweig, 1957.
19. Born, M. and Wolf, E. Principles of optics. Pergamon Press, Oxford, London, New York, Paris, 1964.
20. Gorbatsevich, F. The Ether and Universe. VDM Verlag, Saarbrucken, 2010.
21. Ivanenko, D.D. and Sardanashvily, G.A. Gravitation. Moscow, Editorial URSS, 2004. 200 p. (in Russian).
22. Frankfurt, U.I. Special and General Theory of Relativity. Moscow, Nauka, 1968. 331 p. (in Russian).
23. Maxwell, J.C. Articles and speeches. Moscow, Nauka, 1968. 422 p. (in Russian).
24. Styrkov, E.I. Detecting the influence of the Earth motion on the aberration of electromagnetic waves from a geostationary satellite – a new test of special relativity. In: Space, time, gravity. Proc. of IX Int. Conf. 7-11 August 2006, St. Petersburg. St. Petersburg, “TESSA”, 2007. P. 297-310. (in Russian).
25. Ginzburg, V.L. Radiation of uniformly moving sources – (Vavilov-Cherenkov effect, transition radiation and some other phenomena). Acoustic Journal, Vol. 51, ¹ 1, 2005. P. 24-36. (in Russian).
26. Tyapkin, A.A. Experimental indication of tachyon existence, obtained in the study of Cherenkov radiation. (in Russian).
27. Sommerfeld, A. Gottingen Nachrichten (1904) s. 9, 363; Gottingen Nachrichten (1905) s. 201. (Reference is given by [25]).
28. Mach, E. Mechanics. Historical-critical essay of its development. Izhevsk: Izhevsk Republican Printing Office, 2000. 456 p. (in Russian).
Ïîíÿòèå æå "ôèçè÷åñêîãî âàêóóìà" â ðåëÿòèâèñòñêîé êâàíòîâîé òåîðèè ïîëÿ ïîäðàçóìåâàåò, ÷òî âî-ïåðâûõ, îí íå èìååò ôèçè÷åñêîé ïðèðîäû, â íåì ëèøü âèðòóàëüíûå ÷àñòèöû ó êîòîðûõ íåò ôèçè÷åñêîé ñèñòåìû îòñ÷åòà, ýòî "ôàíòîìû", âî-âòîðûõ, "ôèçè÷åñêèé âàêóóì" - ýòî íàèíèçøåå ñîñòîÿíèå ïîëÿ, "íóëü-òî÷êà", ÷òî ïðîòèâîðå÷èò ðåàëüíûì ôàêòàì, òàê êàê, íà ñàìîì äåëå, âñÿ ýíåðãèÿ ìàòåðèè ñîäåðæèòñÿ â ýôèðå è íåò èíîé ýíåðãèè è èíîãî íîñèòåëÿ ïîëåé è âåùåñòâà êðîìå ñàìîãî ýôèðà.
 îòëè÷èå îò ëóêàâîãî ïîíÿòèÿ "ôèçè÷åñêèé âàêóóì", êàê áû ñîâìåñòèìîãî ñ ðåëÿòèâèçìîì, ïîíÿòèå "ýôèð" ïîäðàçóìåâàåò íàëè÷èå áàçîâîãî óðîâíÿ âñåé ôèçè÷åñêîé ìàòåðèè, èìåþùåãî êàê ñîáñòâåííóþ ñèñòåìó îòñ÷åòà (îáíàðóæèâàåìóþ ýêñïåðèìåíòàëüíî, íàïðèìåð, ÷åðåç ôîíîâîå êîñìè÷åêîå èçëó÷åíèå, - òåïëîâîå èçëó÷åíèå ñàìîãî ýôèðà), òàê è ÿâëÿþùèìñÿ íîñèòåëåì 100% ýíåðãèè âñåëåííîé, à íå "íóëü-òî÷êîé" èëè "îñòàòî÷íûìè", "íóëåâûìè êîëåáàíèÿìè ïðîñòðàíñòâà". Ïîäðîáíåå ÷èòàéòå â FAQ ïî ýôèðíîé ôèçèêå.
|
|
 |
, kg·m2/s2.
,
, kg·m2/s2,